7. Choosing the Appropriate Section Shape

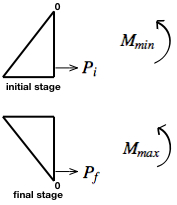
The diagram below illustrates the initial and final conditions, with the simplifying assumption that the concrete can take zero tension.  At the initial stage, the applied moment is M_{min}, and at the final stage, the applied moment is M_{max}.

PS18rev

(1)   \begin{equation*} f_b = -\frac{P_f}{A} - \frac{P_fe}{S_b} + \frac{M_{max}}{S_b},\end{equation*}

where, M_{max} results from all dead and service live loads

Let’s define a new variable: k_t = \frac{S_b}{A}

(2)   \begin{equation*} f_b = -\frac{P_f}{A} - \frac{P_fe}{k_tA} + \frac{M_{max}}{k_tA}\end{equation*}

(3)   \begin{equation*} 0 = -P_fk_t - P_fe + M_{max} \Rightarrow M_{max} = P_f(k_t + e) \end{equation*}

Similarly,

(4)   \begin{equation*} f^t = -\frac{P_i}{A} + \frac{P_ie}{S_t} - \frac{M_{min}}{S_t}, \end{equation*}

where M_{min} typically results from the dead load only

We can define k_b = \frac{S_t}{A}

(5)   \begin{equation*} 0 = -\frac{P_i}{A} + \frac{P_ie}{k_bA} - \frac{M_{min}}{k_bA} \Rightarrow M_{min} = P_ie - P_ik_b = P_i(e-k_b)\end{equation*}

Note: we are neglecting crushing and making the simplification that (f_{tension})_{max} = f_t = 0

So,

(6)   \begin{equation*} P_i \leq \frac{M_{min}}{e-k_b} \text{ and } P_f \geq \frac{M_{max}}{e+k_t} \end{equation*}

We can see that large k_t + k_b \Rightarrow  the section can resist large service loads AND will be able to resist a large prestressing force at the transfer stage (ignoring crushing in either case).

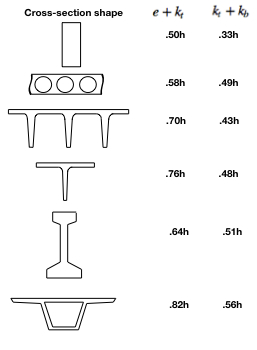
PS19rev

For a continuous beam, or a continuous slab (positive and negative moments), allowable variation may be as important as the  k_t + k_b value.  For example, the hollow-core slab above could be as efficient as the box-girder, in such a scenario.

(7)   \begin{equation*} \frac{M_{max}}{S_b} - \frac{P_f}{A} - \frac{P_fe}{S_b} \leq f_b, \text{ which can be rearranged to yield:} \end{equation*}

(8)   \begin{equation*} P_f \geq \frac{M_{max}-S_bf_b}{e+k_t} \text{ (skipped work)} \end{equation*}

This is what we’ll use for actual design (rather than simply the choosing of section size), as we will see in detail in the following section.

Similarly,

(9)   \begin{equation*} \frac{P_ie}{S_t} - \frac{P_i}{A} - \frac{M_{min}}{S_t} \leq f_t \Rightarrow e \leq k_b + \frac{M_{min} + S_tf_t}{P_i} \text{ (skipped work)} \end{equation*}