Cauchy Equation of Motion

The equation of motion can be expressed in terms of the applied stress, body forces, mass, and acceleration:

(1) $\begin{equation*} \int_S \mathbf{t}dS +\int_V \rho \mathbf{b} dV = \frac{d}{dt}\int_V\rho \mathbf{v}dV \text{ ; } \mathbf{t} = \bm{\sigma} \cdot \mathbf{n} \end{equation*}$

In index notation: $\int_S t_i dS + \int_V \rho b_i dV = \frac{d}{dt}\int_V \rho v_i dV$ ; $t_i=\sigma_{ij}n_j$

(2) $\begin{equation*} \underbrace{\int_S \sigma_{ij}n_j dS}_{\int_V \frac{\partial}{\partial x_j} \sigma_{ij} dV} +\int_V \rho b_i dV = \int_V \rho \frac{dv_i}{dt} dV \end{equation*}$

Eq. 2 is the continuum mechanics version of Newton’s Second Law, often called the balance of linear momentum.

Note that the step from eq. 1 to eq. 2 is not trivial, since both $\rho$ and $dV$ do in fact change with time. The complete derivation, starting with eq. 1 and concluding with eq. 2, is given in Appendix B.2. [Holzapfel] calls this derivation (operation) the “material time derivative of a spatial field.”

Thus,

(3) $\begin{equation*} \int_V (\underbrace{\frac{\partial \sigma_{ij}}{\partial x_j}+\rho b_i - \rho \frac{dv_i}{dt}}_{=0, \text{ since } dV \text{ is arbitrary }})dV=0 \end{equation*}$

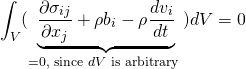
Note that the “localization theorem” states that $\int_{a}^{b} f dx = 0 \longrightarrow f = 0$ , if $a$ and $b$ are arbitrary.

(4) $\begin{equation*} \frac{\partial \sigma_{ij}}{\partial x_j} + \rho b_i = \rho \frac{dv_i}{dt} \end{equation*}$

Eq. 4 are the Cauchy Equations of Equilibrium. It may not yet be clear which term contains the applied forces and which term contains the quantities analogous to “ $k*x$ .” It turns out that the $\sigma_{ij}$ term will contain applied forces and prescribed displacements, along with all of the internal force and displacement quantities that constitute “ $k*x$ ” for the element. When an entire system is analyzed, which includes many elements, the global equation of motion should be satisfied, naturally, so long as the geometry is accurately represented by the elements, the stiffness and strength properties of the material are defined for each element, and the boundary conditions are correctly assigned for each element. There could be other issues that arise as well, due to simplifications inherent (but quite necessary) in the finite element analysis method (FEA), but these issues will be left to texts that cover FEA in detail. In fact, among the aforementioned element-related issues, this text will only cover material elastic stiffness in detail. Material behavior at the limit state (failure) is covered in texts on plasticity, for example, and topics relating to element geometries, prescribed degrees of freedom or prescribed forces at “nodes” (i.e. boundary conditions), or other issues related to the “assembly” of finite elements will be left to texts devoted to the topic of FEA implementation.

$v_i=0$ . then eq. 4 reduces to static equilibrium. An alternative derivation of stress equilibrium, which doesn’t use index notation, can be found in [Ugural].

$\left(\text{static equilibrium: }\frac{\partial \sigma_{i1}}{\partial x_1} + \frac{\partial \sigma_{i2}}{\partial x_2} + \frac{\partial \sigma_{i3}}{\partial x_3}=0 \text{ }(\text{no sum on } i)\right)$

note: This is the basic differential equation used in FEA, though the connection to FEA will not really be clear until we start developing constitutive equations relating stresses and strains (along with the above equation and strain-displacement relationships previously presented).

G. A. Holzapfel, Nonlinear Solid Mechanics: A Continuum Approach for Engineering, Baffins Lane, Chichester, West Sussex PO19 1UD, England: John Wiley & Sons Ltd., 2000.
[Bibtex]

@book{holzapfel,

    address = {Baffins Lane, Chichester, West Sussex PO19 1UD, England},

    author = {Holzapfel, Gerhard A.},
    publisher = {John Wiley \& Sons Ltd.},

    title = {{Nonlinear Solid Mechanics: A Continuum Approach for Engineering}},

    year = {2000}
}

A. C. Ugural and S. K. Fenster, Advanced Mechanics of Materials and Applied Elasticity, Pearson Education, 2011.
[Bibtex]

@book{Ugural,
  title={Advanced {M}echanics of {M}aterials and {A}pplied {E}lasticity},
  author={Ugural, Ansel C and Fenster, Saul K},
  year={2011},
  publisher={Pearson Education}
}

Leave a Reply Cancel reply