Other Rates of Change (Volume and Area)

For completeness, we’ll quickly derive the rate of volume change and the rate of area change:

Volume:
If $dV=ds_1ds_2ds_3$ , where $ds_1$ , $ds_2$ , $ds_3$ are the lengths of the sides of a “box” that is oriented in the principal directions of $\mathbf{D}$ , then:
$\frac{d}{dt}(dV)=\underbrace{\frac{d(ds_1)}{dt}}_{D_{11}ds_1}ds_2ds_3+\underbrace{\frac{d(ds_2)}{dt}}_{D_{22}ds_2}ds_1ds_3+\underbrace{\frac{d(ds_3)}{dt}}_{D_{33}ds_3}ds_1ds_2$

(1) $\begin{equation*} =(D_{11}+D_{22}+D_{33})\underbrace{ds_1ds_2ds_3}_{dV}=D_{kk}dV \text{ note: } tr(\mathbf{D})=D_{kk} \end{equation*}$

Area:
Consider the following time derivative:
$\frac{d}{dt}(dS\mathbf{n}) = \frac{dS}{dt}\mathbf{n}+dS\frac{d\mathbf{n}}{dt}$
where $dS$ is an area (as opposed to $ds_1$ , $ds_2$ , $ds_3$ , which are lengths).

$dS\mathbf{n}$ is known from eq. 2 in Section 2: Volume and Area Change. So, we can take the time derivative. We can also re-write $\frac{d\mathbf{n}}{dt}$ in terms of $D_{nn}$ .

(2) $\begin{equation*} \longrightarrow \frac{d}{dt}(dS)=(\underbrace{D_{kk}}_{\text{sum}}-\underbrace{D_{nn}}_{\text{no sum}})dS \end{equation*}$

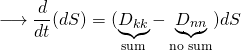
The derivation was skipped here, but can be found in [Holzapfel].

We’ll come back to rates again when we get to rate-form constitutive relationships.

G. Holzapfel, Nonlinear Solid Mechanics, John Wiley & Sons Ltd., England, 2000.
[Bibtex]

@book{Holzapfel,
  title={Nonlinear Solid Mechanics},
  author={Holzapfel, GA},
  year={2000},
  publisher={John Wiley \& Sons Ltd., England}
}

Leave a Reply Cancel reply